Tetrahedrite (Cu₁₂Sb₄S₁₃) is a promising thermoelectric material composed of abundant elements and has a low thermal conductivity because of its complex crystal structure (space 14¯3 m group:) [13]. Lone-pair electrons of the Sb atoms in the tetrahedrite lead to the anharmonic vibration of Cu atoms located inside the triangular plane of the S atoms, which results in a low lattice thermal conductivity [4,5]. Considering the valence and coordination of each element, the charge balance of tetrahedrite can be expressed as Cu22+Cu10+Sb43+S122-S2- [6,7]. To improve the dimensionless figure of merit (ZT), partial substitutions at the Cu, Sb, or S sites have been made with doping elements, including transition metals such as Ni, Co, Fe, and Mn at the Cu site [8-11], Te or As at the Sb site [12,13], and Se at the S site [14].

ZT is influenced by the Seebeck coefficient (α), electrical conductivity (σ), thermal conductivity (κ), and temperature (T) in Kelvin, represented as ZT = α²σκ^-1T. The Seebeck coefficient and electrical conductivity have a trade-off relationship with the power factor (PF = α²σ) because the carrier concentration has the opposite effect on the two parameters [15]. In general, substitutional doping optimizes the carrier concentration, enhancing the PF, or it decreases the thermal conductivity, improving the thermoelectric properties.

Although many doping studies on tetrahedrites have been conducted on Cu sites, few studies have been performed on Sb sites. Bouyrie et al. [12] reported a ZT of 0.80 at 623 K for Cu₁₂Sb_3.39Te_0.61S₁₃, and Lu et al. [16] obtained a ZT of 0.92 at 723 K for Cu₁₂Sb₃TeS₁₃. Kwak et al. [17] reported a ZT of 0.88 at 723 K for Cu₁₂Sb_3.9Bi_0.1S₁₃, and Kumar et al. [18] achieved a ZT of 0.84 at 673 K for Cu₁₂Sb_3.8Bi_0.2S₁₃. In this study, tetrahedrites Cu₁₂Sb_4-ySn_yS₁₃ (y = 0.1-0.4) partially substituted with Sn at the Sb site were synthesized by mechanical alloying, and sintered by hot pressing, and their charge transport and thermoelectric properties were assessed.

Sn-doped tetrahedrites Cu₁₂Sb_4-ySn_yS₁₃ (y = 0.1, 0.2, 0.3, and 0.4) were synthesized by mechanical alloying. Cu (< 45 μm, purity 99.9%, Kojundo Chemical Lab.), Sb (< 150 μm, purity 99.999%, Kojundo Chemical Lab.), Sn (< 35 μm, purity 99.999%, Kojundo Chemical Lab.), and S (< 75 μm, purity 99.99%, Kojundo Chemical Lab.) were weighed to obtain the corresponding stoichiometric compositions. Mechanical alloying was performed at a rotation speed of 350 rpm for 24 h using a planetary ball mill (Fritsch Pulverisette5) in an Ar atmosphere with stainless-steel jars and balls. The synthesized powder was loaded into a graphite mold and subjected to consolidation using hot pressing at 723 K for 2 h under 70 MPa in vacuum. Details of the process conditions have been reported in a previous study [19].

The phases of the synthetic powder and sintered specimens were analyzed using X-ray diffraction (XRD; Bruker D8-Advance) with Cu-Kα radiation. The diffraction patterns were measured in the θ–2θ mode (2θ = 10–90°) with a step size of 0.02°. The lattice constants were analyzed using the Rietveld refinement (TOPAS program). Scanning electron microscopy (SEM; FEI Quanta400) was used to observe the fractured surfaces of the sintered specimens. The composition was analyzed using an energy-dispersive spectrometer (EDS; Bruker Quantax200), where the energy levels of the elements were adopted as Cu-Lα (0.928 eV), Sb-Lα (3.604 eV), Sn-Lα (3.444 eV), and S-Kα (2.309 eV). The Hall coefficient, carrier concentration, and mobility were measured by the van der Pauw (Keithley 7065) method by applying a constant magnetic field of 1 T and a current of 100 mA. The Seebeck coefficient and electrical conductivity were measured using a ZEM-3 (Ulvac-Riko) system in a He atmosphere. The thermal diffusivity and specific heat were measured using the laser flash method with TC-9000H (Ulvac-Riko) equipment, and then the thermal conductivity was estimated. Finally, the PF and ZT were evaluated at temperatures in the range of 323-723 K.

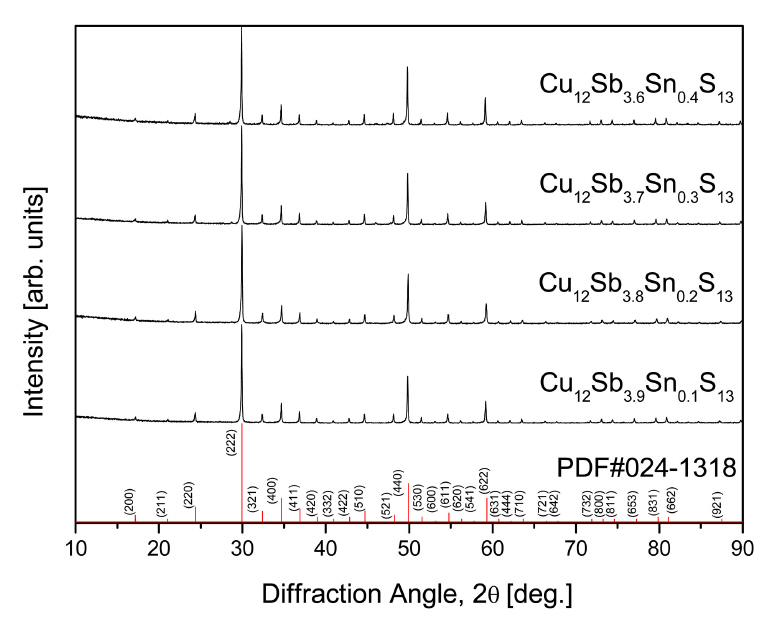
Figure 1 shows the XRD analytical results for the Cu₁₂Sb_4-ySn_yS₁₃ prepared by mechanical alloying and hot pressing. A single tetrahedrite phase (ICDD PDF#024-1318) was identified without secondary phases, and no phase transitions were observed at the doping content range in this study. As Table 1 shows, the lattice constant of Cu₁₂Sb_4-ySn_yS₁₃ increased from 1.0348 to 1.0364 nm as the Sn content increased. In our previous study [7], the lattice constant of undoped tetrahedrite Cu₁₂Sb₄S₁₃ was 1.0327 nm. Thus, the lattice constant was significantly increased by Sn substitution at the Sb site. Tippireddy et al. [20] reported the ionic radii of Cu⁺ (60 pm), Cu²⁺ (57 pm), Sb³⁺ (76 pm), Sn⁴⁺ (69 pm), and Cu²⁺ (118 pm). Hansen et al. [21] suggested that Sn⁴⁺ can be doped into the Cu sites when no other divalent transition elements are present in the tetrahedrite system, suggesting that Cu²⁺ can be substituted at the Sb³⁺ site, and Sn⁴⁺ can be substituted for Cu⁺ and/or Cu²⁺. Therefore, in this study, as the Sn content increased, the lattice constant increased.

Figure 2 shows SEM images of the fractured surfaces of Cu₁₂Sb_4-ySn_yS₁₃. No remarkable difference in microstructure was observed based on Sn content, and each element was homogeneously distributed, as confirmed by EDS elemental mapping. As Table 1 shows, all the specimens reached relatively high densities of 99.5%-100.0%, and the actual composition was similar to its nominal composition within the error ranges of the EDS analysis. Therefore, in this study, mechanical alloying and hot pressing resulted in the successful preparation of homogeneous and dense Sn-doped tetrahedrite compounds.

Figure 3 shows the carrier concentration and mobility of the Cu₁₂Sb_4-ySn_yS₁₃. As the Sn content increased, the carrier concentration decreased from 1.28 × 10¹⁹ to 1.57 × 10¹⁸ cm^-3. Sn⁴⁺ substituted at the Sb³⁺ site provided excess electrons, which reduced the carrier (hole) concentration due to charge compensation. Considering the ionic radii claimed by Tippireddy et al. [20] when Sn is doped in the tetrahedrite, either Sn⁴⁺ should be substituted at the Cu⁺ or Cu²⁺ site or Cu²⁺ at the Sb³⁺ site to increase the lattice constant, as shown in this study. However, if the entirety of the doped Sn is substituted at the Sb³⁺ site into a Cu²⁺ state, we should observe a reduction in carrier (hole) concentration. Therefore, this study interpreted that Sn⁴⁺ was partially substituted at the Cu⁺/Cu²⁺ sites and/or Sn⁴⁺ was also partially substituted at the Sb³⁺ site. This contributed to an increase in the lattice constant and a decrease in the carrier concentration. When y ≤ 0.3, the mobility slightly increased with increasing Sn content but decreased when y = 0.4. The reason the mobility of Cu₁₂Sb_3.6Sn_0.4S₁₃ decreased is uncertain, but it was assumed to be because of the change in the conduction mechanism.

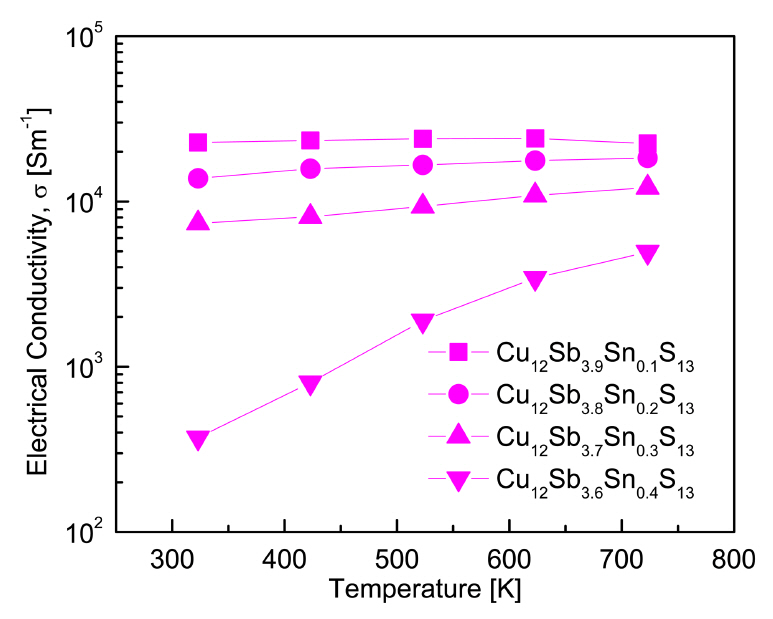
The electrical conductivities of the Cu₁₂Sb_4-ySn_yS₁₃ samples are shown in Fig 4. The electrical conductivities (σ) of semiconductors are typically affected by the carrier concentration (n) and mobility (μ), which is represented as σ = neμ (e: electronic charge) [22]. For y = 0.1, the specimen in our study exhibited minimal temperature dependence up to 623 K but showed a slight decrease at temperatures above 623 K, indicating degenerate semiconductor behavior. For y = 0.2-0.3, the electrical conductivity slightly increased with increasing temperature. However, in the case of y = 0.4, the electrical conductivity showed a strong positive temperature dependence, indicating non-degenerate semiconductor behavior. Thus, it was determined that the electronic conduction mechanism transitioned from a degenerate to a non-degenerate state when the tetrahedrite was doped with Sn. At a constant temperature, the electrical conductivity decreased as the Sn content increased. This is in good agreement with the decrease in the carrier concentration as the Sn doping level increased, as shown in Fig 3.

Cu₁₂Sb_3.9Sn_0.1S₁₃ exhibited the highest electrical conductivity of (2.24-2.40) × 10⁴ Sm^-1 at temperatures between 323-723 K. For the electrical conductivity of undoped Cu₁₂Sb₄S₁₃, Kim et al. [19] reported (2.17-3.03) × 10⁴ Sm^-1 at 323-723 K, and Pi et al. [23] obtained (1.10-1.90) × 10⁴ Sm^-1 at 323-723 K. Kwak et al. [17] reported that the electrical conductivity increased with increasing Bi content and positive temperature dependence in the cases of y ≤ 0.3 for Cu₁₂Sb_4-yBi_yS₁₃ (y = 0.1-0.4): as a result, from (2.20-3.12) × 10⁴ Sm^-1 at 323 K to (2.95-3.25) × 10⁴ Sm^-1 at 723 K. However, when y = 0.4, the electrical conductivity decreased because of the formation of a secondary phase (skinnerite Cu3SbS3). Conversely, Bouyrie et al. [12] reported negative temperature dependence of the electrical conductivity for Cu₁₂Sb_4-xTe_xS₁₃ (x = 0.5-2.0): from (9.09-0.36) × 10⁴ Sm^-1 at 300 K to (4.54-0.42) × 10⁴ Sm^-1 at 700 K. Tippireddy et al. [20] reported that the electrical conductivity decreased with increasing Sn content and there was a negative temperature dependence for Cu₁₂Sb_4-xSn_xS₁₃ (x = 0.25-1): from (5.46-3.95) × 10⁴ Sm^-1 at 373 K to (4.80-3.09) × 10⁴ Sm^-1 at 673 K.

Figure 5 shows the Seebeck coefficients of the Cu₁₂Sb_4-ySn_yS₁₃ samples. The Seebeck coefficient values of all specimens were positive, indicating that the major carriers were holes of p-type semiconductors. The Seebeck coefficient of a ptype semiconductor is expressed as α = (8/3) π² k_B²m*Te^-1h^-2 (π/3n)^2/3, where k_B is the Boltzmann constant, m* is the effective carrier mass, and h is the Planck constant [24]. In our study, as the temperature increased, the Seebeck coefficient increased when y ≤ 0.3, but when y = 0.4, the Seebeck coefficient decreased. In general, when the temperature was higher than a certain temperature (i.e., intrinsic transition temperature), the carrier concentration increased rapidly, and the Seebeck coefficient decreased. This was related to the temperature dependence of the electrical conductivity, as shown in Fig 4.

Accordingly, the intrinsic transition seemed to occur at temperatures below 323 K for Cu₁₂Sb_3.6Sn_0.4S₁₃. At a constant temperature, the Seebeck coefficient increased as the Sn content increased. Thus, Cu₁₂Sb_3.6Sn_0.4S₁₃ exhibited a maximum Seebeck coefficient of 270-238 μVK^-1 at temperatures of 323-723 K. In the case of undoped Cu₁₂Sb₄S₁₃, Kim et al. [19] reported a Seebeck coefficient of 134-183 μVK^-1 at 323-723 K, and Pi et al. [23] obtained 155-195 μVK^-1 at 323-723 K. Kwak et al. [17] reported that the Seebeck coefficient decreased as the Bi content increased for Cu₁₂Sb_4-yBi_yS₁₃ (y = 0.1-0.4), and obtained the highest Seebeck coefficient of 153-186 μVK^-1 at 323-723 K for Cu₁₂Sb_3.9Bi_0.1S₁₃. However, Bouyrie et al. [12] found that the Seebeck coefficient increased with increasing Te content for Cu₁₂Sb_4-xTe_xS₁₃ (y = 0.50-1.75). Thus, the highest Seebeck coefficient of 192-264 μVK^-1 was obtained at 300-700 K for Cu₁₂Sb_2.25Te_1.75S₁₃. Tippireddy et al. [20] reported that the Seebeck coefficient decreased with increasing Sn content for Cu₁₂Sb_4-xSn_xS₁₃ (x = 0.25-1), reaching a maximum value of 183 μVK^-1 at 673 K for Cu₁₂Sb_3.65Sn_0.35S₁₃.

Figure 6 presents the PF of Cu₁₂Sb_4-ySn_yS₁₃. Based on the temperature dependences of the electrical conductivity and Seebeck coefficient shown in Figs 4 and Fig 5, respectively, the PF increased with increasing temperature. Because both the Seebeck coefficient and electrical conductivity are affected by the carrier concentration, as the Sn content increased, the carrier concentration (i.e., electrical conductivity) decreased, which decreased the PF. In this study, a maximum PF of 0.38-0.73 mWm^-1K^-2 was obtained at 323-723 K for Cu₁₂Sb_3.9Sn_0.1S₁₃. For undoped Cu₁₂Sb₄S₁₃, Kim et al. [19] and Pi et al. [23] reported 0.40-0.95 mWm^-1K^-2 and 0.26-0.72 mWm^-1K^-2 at 323-723 K, respectively. Kwak et al. [17] obtained a high PF of 1.02 mWm^-1K^-2 at 723 K for Cu₁₂Sb_3.9Bi_0.1S₁₃, and Bouyrie et al. [12] reported 0.95 mWm^-1K^-2 at 700 K for Cu₁₂Sb_3.5Te_0.5S₁₃. Tippireddy et al. [20] achieved a very high PF of 1.30 mWm^-1K^-2 at 673 K for Cu₁₂Sb_3.65Sn_0.35S₁₃, which was anomalously higher than in other studies on tetrahedrite at all temperatures examined.

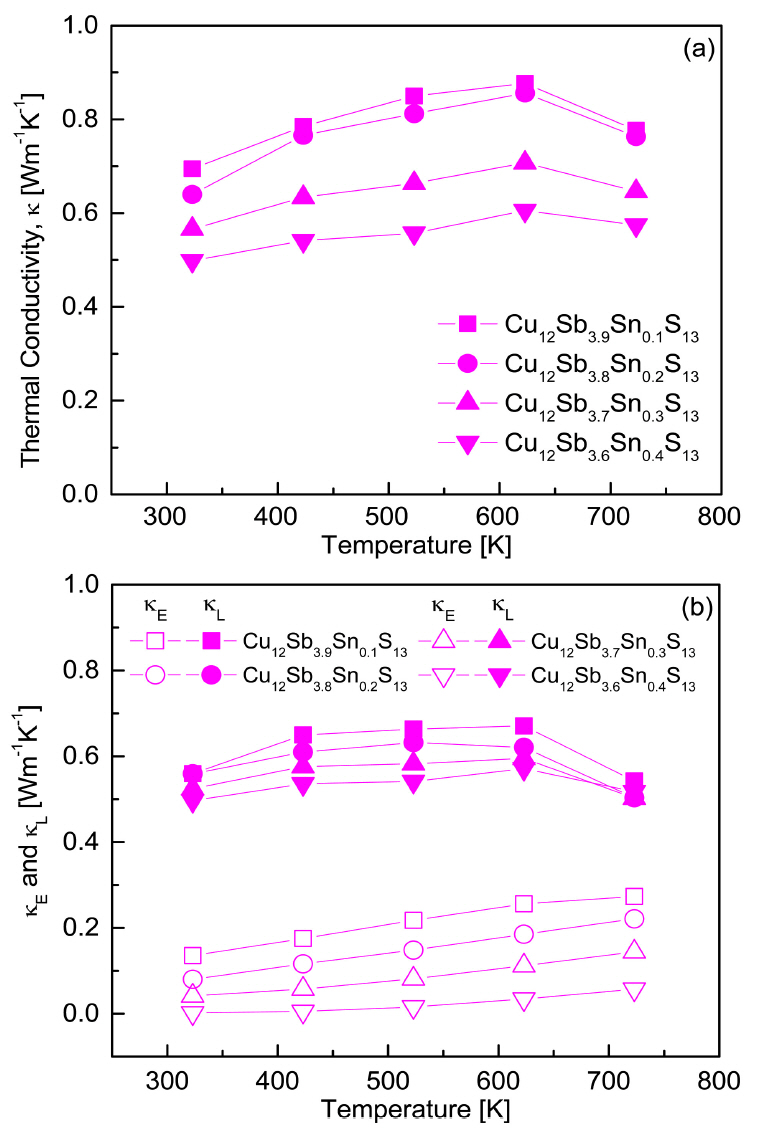
Figure 7 shows the thermal conductivities of the Cu₁₂Sb_4-ySn_yS₁₃ samples. From the relationship κ = κ_E + κ_L, the lattice thermal conductivity (κ_L) was obtained by subtracting the electronic thermal conductivity (κ_E) from the total thermal conductivity (κ). The electronic thermal conductivity was calculated using the Wiedemann–Franz law (κ_E = LσT, L: Lorenz number) [25], where the Lorenz number was estimated using the formula L[10^-8V²K^–2]= 1.5+exp(–|α|/116) [26,27]. Figure 7(a) shows the total thermal conductivity of Cu₁₂Sb_4-ySn_yS₁₃. As the temperature increased, the total thermal conductivity increased and then decreased at temperatures above 623 K due to the influence of the lattice thermal conductivity. At a constant temperature, the thermal conductivity decreased as the Sn content increased. Cu₁₂Sb_3.6Sn_0.4S₁₃ exhibited the lowest thermal conductivity of 0.49-0.60 mWm^-1K^-1 at 323-723 K, which was lower than the 0.72-0.82 mWm^-1K^-1 and 0.65-0.78 mWm^-1K^-1 of undoped Cu₁₂Sb₄S₁₃ as reported by Kim et al. [19] and Pi et al. [23], respectively. Kwak et al. [17] obtained a minimal thermal conductivity of 0.77-0.75 mWm^-1K^-1 at 323-723 K for Cu₁₂ Sb_3.6Bi_0.4S₁₃. Tippireddy et al. [20] reported a minimum thermal conductivity of 0.61-0.79 mWm^-1K^-1 at 373-673 K for Cu₁₂Sb_3.75Sn_0.25S₁₃ and suggested that localized energy caused by lone-pair electrons on Sb scattered the high-velocity acoustic phonons and hybridized with the acoustic dispersions, leading to a reduction in thermal conductivity. Therefore, this study confirmed that the thermal conductivity decreased when Sn was substituted for Sb.

As Fig 7(b) shows, the electronic thermal conductivity increased with increasing temperature but decreased with increasing Sn content, resulting in a minimum electronic thermal conductivity of 0.002-0.057 mWm^-1K^-1 at 323-723 K for Cu₁₂Sb_3.6Sn_0.4S₁₃. This was much lower than in other studies: 0.19-0.34 mWm^-1K^-1 at 323-723 K for Cu₁₂Sb₄S₁₃ [19], 0.19-0.40 mWm^-1K^-1 at 323-723 K for Cu₁₂Sb_3.6Bi_0.4S₁₃ [17], and 0.27-0.34 mWm^-1K^-1 at 373-673 K for Cu₁₂Sb₃SnS₁₃ [20]. In this study, the lattice thermal conductivity decreased as the Sn content increased, resulting in a minimum lattice thermal conductivity of 0.49-0.57 mWm^-1K^-1 at 323-723 K for Cu₁₂Sb_3.6Sn_0.4S₁₃. Kim et al. [19] reported a lattice thermal conductivity of 0.43-0.59 mWm^-1K^-1 at 323-723 K for Cu₁₂Sb₄S₁₃. Kwak et al. [17] obtained a minimum lattice thermal conductivity of 0.58-0.35 mWm^-1K^-1 at 323-723 K for Cu₁₂Sb_3.6Bi_0.4S₁₃, and Tippireddy et al. [20] achieved the lowest lattice thermal conductivity of 0.23-0.32 mWm^-1K^-1 at 373-673 K for Cu₁₂Sb_3.75Sn_0.25S₁₃.

Figure 8 shows the Lorenz numbers of Cu₁₂Sb_4-ySn_yS₁₃. Generally, the Lorenz number falls in the range of (1.45-2.44) × 10^-8 V²K^-2 [28]. Higher and lower Lorenz numbers indicate degenerate semiconductor or metallic behavior and non-degenerate semiconductor behavior, respectively. In this study, the Lorenz number decreased with increasing Sn content, which was considered to be a transition from degenerate to non-degenerate states. Cu₁₂Sb_3.9Sn_0.1S₁₃ showed the highest Lorenz number of (1.82-1.71) × 10^-8 V²K^-2, but Cu₁₂Sb_3.6Sn_0.4S₁₃ exhibited the lowest Lorenz number of (1.59-1.62) × 10^-8 V²K^-2 at 323-723 K. Similar Lorenz numbers were reported by Kim et al. [19] and Pi et al. [23], namely, (1.71-1.81) × 10^-8 V²K^-2 and (1.76-1.69) × 10^-8 V²K^-2 at 323-723 K for Cu₁₂Sb₄S₁₃, respectively, and by Kwak et al. [17], namely, 1.88 × 10^-8 V²K^-2 at 323 K for Cu₁₂Sb_3.7Bi_0.3S₁₃.

The ZT for Cu₁₂Sb_4-ySn_yS₁₃ is shown in Fig 9. As the temperature increased, the ZT increased as the PF increased. Although the thermal conductivity decreased as the Sn content increased, the decrease in the PF was dominant. Therefore, the increase in Sn doping level could not further improve the ZT.

A maximum ZT of 0.66 was obtained at 723 K for the Cu₁₂Sb_3.9Sn_0.1S₁₃. The ZT values of the Sb-site-doped tetrahedrites were compared. Bouyrie et al. [12] reported that a ZT of 0.65 at 623 K for Cu₁₂Sb_3.5Te_0.5S₁₃ was generated by the multi-step annealing and spark-plasma sintering (SPS) process. Kwak et al. [17] obtained a ZT of 0.88 at 723 K for Cu₁₂Sb_3.9Bi_0.1S₁₃ prepared by mechanical alloying and hot pressing. Tippireddy et al. [20] achieved a ZT of 0.96 at 673 K for Cu₁₂Sb_3.65Sn_0.35S₁₃ fabricated by the annealing-milling-SPS process.

In this study, Sn-doped tetrahedrites Cu₁₂Sb_4-ySn_yS₁₃ (0.1 ≤ y ≤ 0.4) were synthesized by mechanical alloying and sintered by hot pressing. A single phase of tetrahedrite was obtained without secondary phases or residual elements without post-annealing treatment. The lattice constant increased as the Sn doping content increased, and Sn was substituted at the Sb site. As the Sn content increased, the carrier concentration decreased due to the charge compensation resulting from the excess supply of electrons. As the Sn content increased, mobility tended to increase when y ≤ 0.3, but decreased rapidly when y = 0.4, which was presumed to be caused by a change in the conduction mechanism, from a degenerate to a non-degenerate state. The electrical conductivity increased as the Sn content decreased, and the temperature dependence was minimal when y = 0.1-0.3. However, the electrical conductivity of the specimen with y = 0.4 increased rapidly as the temperature increased, similar to the behavior of a non-degenerate semiconductor. The Seebeck coefficient increased as the Sn content increased. For y = 0.1-0.3, the Seebeck coefficient increased as the temperature increased, but when y = 0.4, it decreased. This was because the carrier concentration increased rapidly at temperatures above the intrinsic transition temperature. The PF increased as the temperature increased, but it decreased with increasing Sn doping content. The PF was dominated by the influence of electrical conductivity. When the Sn content increased at a constant temperature, both the lattice and electronic thermal conductivities decreased. The Lorenz number decreased as the Sn content increased, changing from a degenerate to a non-degenerate state. The maximum ZT of 0.66 was obtained at 723 K for Cu₁₂Sb_3.9Sn_0.1S₁₃.